This is the article in which the trace formula first appeared:

A. Selberg, "Harmonic analysis and discontinuous groups in weakly symmetric Riemannian spaces with applications to Dirichlet series", Journal of the Indian Mathematical Society 20 (1956) 47-87.

General information on the importance and context of the trace formula can be found here.

What is often referred to in the literature as "the Selberg trace formula" is actually a particular case of the general formula Selberg proves in this paper.

The general formula he develops in section 2 applies to a Riemannian manifold S of any dimension, together with a locally compact group of isometries G acting on S, and a discrete subgroup of G.

In section 3, he treats the special case where S is the hyperbolic plane. G and its subgroup are chosen appropriately so that the formula relates to compact Riemannian surfaces (which are well-known to be expressible as quotients of the hyperbolic plane).

This is the formula as given on p.74 of Selberg's paper:

Put very simply, this is a relationship between the harmonic spectrum of eigenvalues on a compact Riemann surface and the set of lengths of periodic geodesics on the surface. We are going to look at the formula in some detail.

The left-hand side is effectively a sum over the Laplacian spectrum (i.e. the harmonic frequencies) of the compact Riemannian surface. The r_i are related to the eigenvalues of the Laplace-Beltrami operator (invariant Laplacian) on the quotient surface according to a simple rule.

HEJHAL (p.455) seems to suggest the following relationship with the eigenvalues of the Laplace-Beltrami operator: lambda_n = 1/4 + r_n². Selberg himself (p.74) says something similar, but there's a confusing y².

Both left and right hand sides can be understood as distributions, where h is the 'test-function' being acted on (the g in the final term of the RHS is just an integral transform of h).

Hejhal explains the relationship between g and h on p.454-455:

h(r) = Integral_-inf^+inf g(u)e^irudu

with inversion formula:

g(r) = (1/2pi)Integral_-inf^+inf h(u)e^-irudr

h is meant to satisfy three conditions:

• Symmetry: h(r) = h(-r)
• Analyticity: h(r) is regular analytic in a strip |Im r| < 1/2 + epsilon, where epsilon > 0
• Boundedness: h(r) = O((1 + |r|²) ^-1-epsilon.

Recall that the big 'O' notation means that h(r) is less than some constant multiple of the argument.

The three terms on the right-hand side all originate with a single sum on the right-hand side of Selberg's general trace formula. This is a sum over all primitive conjugacy classes in the subgroup of isometries. Conjugacy is a simple equivalence relation on the isometry subgroup, defined as follows: Two isometries G₁ and G₂ are said to be conjugate (written G₁ ~ G₂) if for some H in the subgroup we have

G₁ = HG₂H^-1

This relation allows us to partition the isometry subgroup into equivalence classes of mutually conjugate isometries. Below we will see that conjugacy has a geometric significance relating to the homotopy of the surface.

NOW EXPLAIN ABOUT THE 'PRIMITIVE' BIT. IS THE ISOMETRY SUBGROUP HERE GAMMA? REFER TO IT BY NAME...

We can split the sum into three parts, corresponding to the identity (a conjugacy class unto itself), hyperbolic conjugacy classes and elliptic conjugacy classes. D. Hejhal explains in his article

"The Selberg trace formula and the Riemann zeta function", Duke Mathematics Journal 43 (1976) p.459

why there can be no parabolic conjugacy classes in this situation. He also explains why the fundamental polygon D has finite area, a quantity which appears in the above formula as A(D).

EXPLAIN ABOUT THE HYPERBOLIC,ELLIPTIC,PARABOLIC BREAKDOWN. BUT FIRST YOU NEED TO BACK UP AND RUN THROUGH THE BASICS:
(1) the hyperbolic plane (various models, links, etc.)
(2) isometries on the hyperbolic plane
(3) discrete subgroups of isometries 'tiling' the hyperbolic plane
(4) fundamental polygons (with illustrations, etc.)
(5) identificiation of edges to produce genus n Riemann surfaces (with constant negative curvature, you should stress)
(6) 'straight lines' on the hyperbolic plane become geodesics on these surfaces

THIS should put things in context.

PRESUMABLY THAT AREA OF THE FUNDAMENTAL POLYGON IN THE FORMULA IS HYPERBOLIC AREA?

The second and third terms of the right-hand side are sums over primitive elliptic and hyperbolic conjugacy classes, respectively: The {R} refers to conjugacy classes of elliptic isometries, and {P} to conjugacy classes of hyperbolic isometries. Note that Hejhal's 1976 paper (mentioned above) presents a simpler version where there are no elliptic conjugacy classes.

EXPLAIN HOW/WHY WE CAN ASSUME THERE ARE NO ELLIPTIC CLASSES. EXPLAIN nu, sigma, chi, matrix representation, M(?) GO INTO DETAIL AS TO HOW EXACTLY THESE THREE DISPARATE TERMS ARISE FROM A SINGLE SOURCE.

The N{P} in the third term are crucial here. They are the norms of primitive conjugacy classes of hyperbolic isometries. Roughly speaking the norm measures the factor by which such an isometry 'dilates' the hyperbolic plane (which is the universal covering surface of the compact Riemann surface in question). These norms are closely related to the lengths of primitive geodesics on the surface and, in the setting of (quantum) chaos, the periods of primitive orbits in certain flows.

The following is Appendix D from A. Voros and N.L. Balasz, "Chaos on the pseudosphere", Physics Reports 143 no. 3.
 

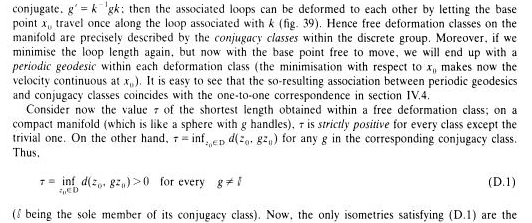
RUN THROUGH this carefully, and add any necessary comments. REFER BACK to A. Strombergsson's comments to you - contact him with questions if you're stuck.

INCLUDE LINKS TO BASIC RESOURCES ON HYPERBOLIC PLANE, ETC. Use Balasz and Voros extensively. PICTURESQUE METAPHOR - something you hit, makes a noise, etc., bits of elastic - does this hold? Stress the nature of these RIEMANN SURFACES...constant curvature -1, impossible to embed in Euclidean space...EXACT DEFINITIONS...

OTHER PROBLEMS/CONCERNS?