

"I sometimes have the feeling that the number system is comparable
with the universe that the astronomer is studying...The number system
is something like a cosmos."
In recent years, a rapidly expanding body of
work has been making unexpected, seemingly unrelated connections between
the mysterious
distribution of prime numbers and various branches of physics. Note that in
general, mathematics 'informs' physics, but not vice versa. That is,
mathematicians have traditionally been able to provide physicists
with useful insights and techniques, but this has been largely a
one-way process. What we are considering here is the reverse
process, where insights and techniques derived from physics are
shedding new light on pure mathematical (in particular, number
theoretical) concerns. The following pages are an attempt to
document and archive this material as comprehensively as possible:
number theory and physics archive
As far as I am aware, no general explanation has been put forward as to why this should be happening – i.e. why elaborate concepts, structures and phenomena developed and studied by physicists, such as thermodynamic partition functions, quantum harmonic oscillators, spontaneous symmetry breaking, 1/f noise, Hagedorn catastrophes, pion-nucleon scattering, The Fokker–Planck equation, the Wiener–Khintchine duality relation, etc. should all be somehow relevant to the purest of pure mathematical structures – the sequence of prime numbers.
However, some months before I became aware of any of the various material compiled in the above-mentioned archive, an image emerged out of my dream-consciousness, and turned into one of the strangest ideas ever to have entered my mind:
In some previously unexplored context, the familiar 'shape' of the sequence of prime numbers is the result of a kind of dynamic or evolutionary process.
Although I was aware that this might be completely meaningless, the idea had such a profound effect on me that I attempted to find a rigorous mathematical framework in which it could perhaps be given some meaning.
In the process, I gradually discovered the various material mentioned above, and found it rather encouraging. For suppose we imagine that there is some meaningful content to my unorthodox speculation, i.e there does exist some sort of mysterious dynamics underlying the distribution of prime numbers. We might then expect to find certain 'clues' as to the nature of the dynamics hidden in the subtleties of the prime distribution. Perhaps this is what is happening.
The following page documents an initial attempt to provide a coherent
mathematical foundation for the concept. I have gone through phases of being no
longer sure what to think about this idea, but have felt that it is worth leaving the
document online for anyone who might be interested.
'prime evolution' notes
In late August 2004, I was able to announce a promising new development, but it is impossible to tell whether the relevance of this goes beyond a superficial similarity of ideas.
These ideas may turn out to be of more psychological than mathematical interest. I feel that at the very least I picked up on some kind of 'resonance' with an important set of ideas emerging in the mathematical sciences, even if the form into which my mind translated this may have been somewhat naïve.
The following rather extraordinary preprint, which I discovered recently (April 2001), although not directly related, may turn out to be relevant here:
I.V. Volovich, "Number theory as the ultimate physical theory", p-Adic Numbers, Ultrametric Analysis and Applications 2 (2010) 77–87
You are invited to send me any comments, questions, or criticism. My original hope was
that the notes would eventually attract the attention of researchers
appropriately specialised to either make use of the ideas involved, or to
demonstrate conclusively that they are meaningless.
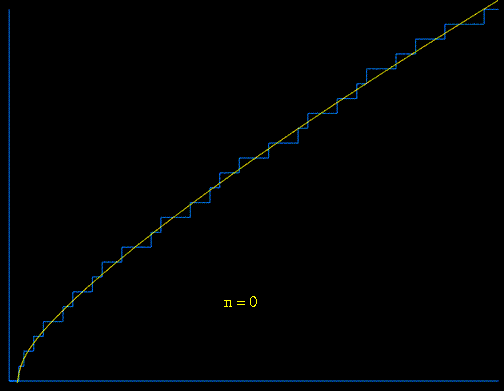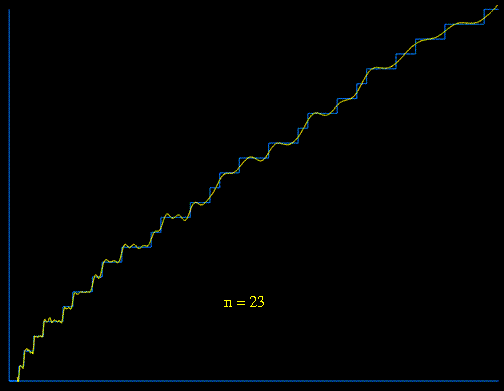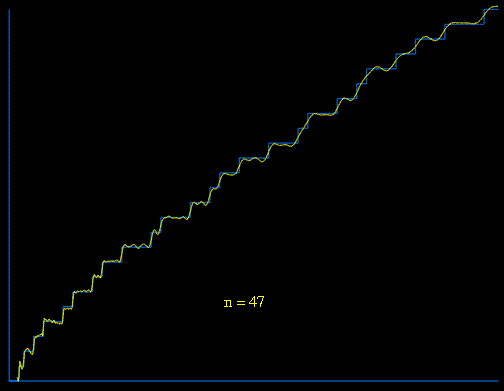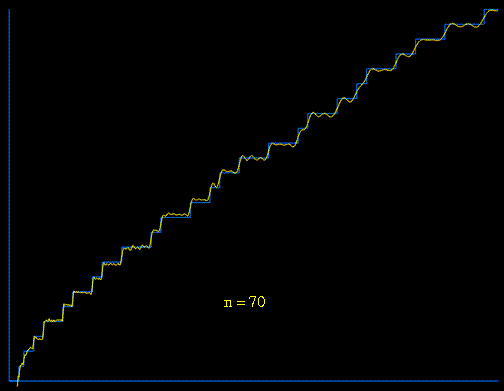
This animation depicts the approximation of the prime counting function π(x) = number of primes <x (shown in blue) using the first seventy pairs of nontrivial zeros of the Riemann zeta function in a variant of von Mangoldt's explicit formula. At each step, the current function (shown in yellow) is modified by adding a waveform whose frequency and amplitude are related to the next pair of nontrivial (complex) zeros in a very simple and direct way.
The horizontal animation at the top of this page is based on the gradient of the yellow
curve, so that the primes emerge from a homogeneous field as 'points of light'. It is the closest thing I have
been able to find within existing mathematical theory to my aforementioned 'inner perception'.
Also available is a similar animation depicting the
emergence of
Chebyshev's function ψ(x) which is a logarithmically-weighted
prime counting function of great importance (for example in
the proof of the prime number theorem.)
number theory and physics archive inexplicable secrets of creation
prime numbers: FAQ and resources home