Abstract: The form of the prime number distribution function has withstood the efforts of all the mathematicians that have considered it. Here we address this problem with the tools of chaotic dynamics and find that, from a physical point of view, this distribution function is chaotic.

[Commentary]

The article begins:

"A classical and long standing problem in number theory is the behaviour of the prime number distribution [1].

Several attempts to find a regular pattern for the prime distribution have been made in the past [2] and, to our knowledge, none of them was successful.

From a strictly mathematical point of view, this problem was extensively studied and still remains unsolved. However, some statistical results have been obtained, e.g. the fractions of intervals which contains exactly k primes follow a Poisson distribution [3]."

From a physical point of view we thought that if we find that this distribution is chaotic, some non-rigorous answers can be provided."

They go on to discuss the connection with quantum chaology, and the study of the Riemann zeta function by Berry, et.al. in the search for a model of quantum chaos [4], and a possible proof of the Riemann hypothesis, based on the "spectral conjecture" of Hilbert and Pólya. The GUE hypothesis is also mentioned.

They continue:

"Furthermore, as there is an increasing interest in applying number theory to chaotic dynamics [6], we think that it is worthwhile to look into the older number theory problem with the tools provided by classical chaotic dynamics.

The evolution of the power spectrum and Liapunov exponents lambda_Iare the most elementary tests to be applied to a series of numbers generated by an unknown dynamics in order to search for some hidden regularity. If at least one of the lambda_I is positive, we know the underlying dynamics is chaotic [7]."

Rather than analysing the sequence of primes itself, the authors consider the difference between the prime counting function 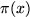and the analytic approximation function R(x) given by Riemann. This difference function D(x) shows how the actual distribution of primes fluctuates around its analytic density. In some sense it is a more direct representation of the "behaviour" of the primes than the more obvious 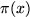. They include a graph of D(x) as their Figure 1.

^{Fig.1. Evolution of the D(x) function; N is the natural number succession}

Figure 1 shows the evolution of D(x) and we analyze its seemingly erratic behaviour by calculating its power spectrum and Liapunov exponent."

Figure 2 shows the corresponding power spectrum. They observe a broad band at low frequencies, and point out that it is quite a strong (necessary but not sufficient) indication of chaotic behaviour.

[Figure 3 to be inserted here]

Figure 3 shows the variation of the largest Liapunov exponent with the size of the analyzed succession. The authors observe that it is unequivocally positive in all the range and, after an initial increase, a wide plateau is reached with a convergent value of 0.11 after nearly 20,000 points. They calculated this with the method of Eckmann, et. al. [9]. It turns out that this method also gives a minimal embedding dimension of for the unknown underlying classical dynamic system.

The other Liapunov exponents are 0.00, -0.04, and -0.14 to give a sum of -0.07. The authors safely conclude that their statistics are not sufficient to conclude reliably whether the system in question is conservative or dissipative.

To conclude:

"Therefore we can safely conclude that a regular pattern describing the prime number distribution cannot be found. Also, from a physical point of view, we can say that any physical system whose dynamics is unknown but isomorphic to the prime number distribution has a chaotic behaviour."

References:

[1] M.R. Schroeder, Number theory in science and communications, Springer series in information sciences (Springer, Berlin, 1986).

[2] M. Gardner, Scientific American, 210(3) (1964) 120.

[3] P.X. Gallagher, Mathematika 23 (1976) 4.

[4] M.V. Berry, Proceedings of the Royal Society A 413 (1987) 183; in Springer lecture notes in physics, Vol. 263. Quantum chaos and statistical nuclear physics, eds. T.H. Seligman and H. Nishioka (Springer, Berlin, 1986) p.1.

[6] I. Percival and F. Vivaldi, Physica D 25 (1987) 105.

[7] J.-P. Eckmann and D. Ruelle, Rev. Mod. Physics 57 (1985) 617; H.G. Schuster, Deterministic Chaos (Physik-Verlag, Weinheim, 1984).

[9] J.-P. Eckmann, S. Oliffson Kamphorst, D. Ruelle and S. Ciliberto, Physical Review A 34(1986) 4971.